Medians of a Triangle
Medians of a Triangle: Overview
This Topic covers sub-topics such as Median of Triangles, Centroid of a Triangle, Circumcentre, Incentre of Triangle and, Basic Properties of Medians of Triangles
Important Questions on Medians of a Triangle
Define centroid of a triangle. Write its properties and the formula for centroid of a triangle.
Define median of a triangle and write its properties.
Write the difference between the median and the altitude of a triangle.
The incenter of a triangle is the point of intersection of all the perpendicular bisectors of the sides of a triangle.
The incentre always lies inside the triangle.
Prove that the incentre of a triangle is equidistant from its sides.
If is the centroid of a and is any other point in the plane, then is equal to
The medians divide the triangle into smaller triangles of equal area.
Every triangle has medians.
Given is the median, which of the following statements is false?
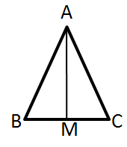
is an isosceles triangle with . and are two medians of the triangle. Then ........
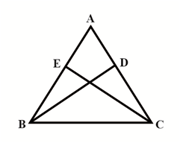
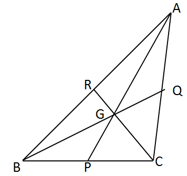
Point G is the centroid of .
If then ?
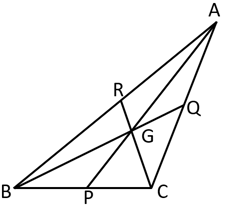
Point is the centroid of . If , then find .
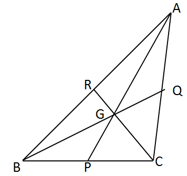
Point G is the centroid of .
If then ?
is a median of the triangle . If then find .
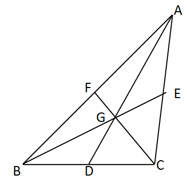
is the centroid of triangle and . If the length of is then find .
The centroid is the point of concurrency of the _____of a triangle.
Which of the following describes a median of a triangle?
In the median and passes through the point . If then find (in ) (correct up to one decimal place)
In the median and passes through the point . If then, find (in ).
